오랜만에 풀어보는 오일러 프로젝트 하나.
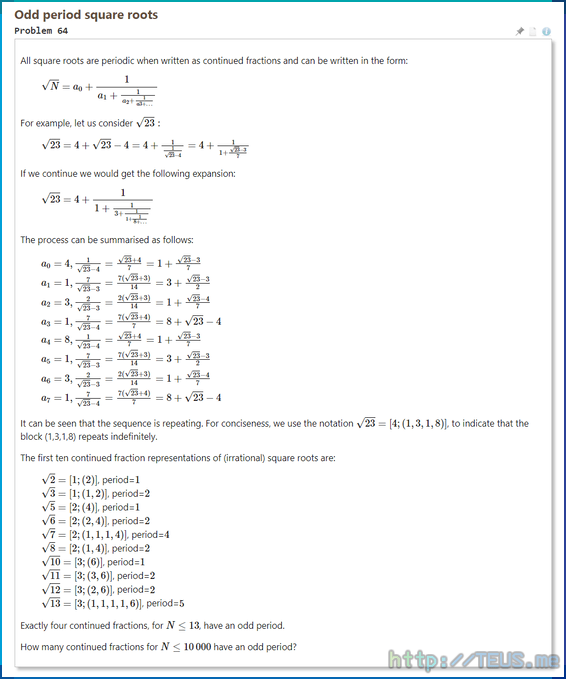
제곱근을 연분수로 전개하면 나오는 값은 순환하게 되는데, 이 값이 홀수 개인 경우를 찾는 문제다.
이 문제는 프로그래밍 난이도 보다는 수학적인 의미를 이해하는 쪽이 더 어려운 문제.
\( \frac { denom } { \sqrt {N} - minuend } \) 형태의 항의 다음 항을 계산하는 식만 찾으면 다음은 간단하다.
\( \frac {denom} { \sqrt {N} - minuend } = \frac {denom ( \sqrt{N} + minuend ) } { N - minuend ^ 2 } = \frac { \sqrt {N} + minuend } {(N - minuend ^ 2) / denom} \)가 되는데, 이 식의 정수해가 다음 항이 된다.
즉, \( a_{n} = \lfloor \frac { \sqrt {N} + minuend } {(N - minuend ^ 2) / denom} \rfloor \) 가 되는데, 여기서 \( \sqrt {N} \)의 정수 파트만 계산하면 나머지는 간단하다.
\( \frac {denom ( \sqrt{N} + minuend ) } { N - minuend ^ 2 } \)는 \( a_{n} + \frac {denom \sqrt{N}+denom \cdot minuend - a_{n}(N - minuend ^ 2 )}{ N - minuend ^ 2 } = a_{n} + \frac {\sqrt{N} - ( a_{n} (N-minuend ^ 2 )/denom - minuend)}{( N - minuend ^ 2 ) / denom } \)로 정리된다.
해를 찾는 과정에서 \( \sqrt{N} \)는 정수 파트만 필요하므로 아래 함수를 사용하면 더 효율적이다.
unsigned isqrt(unsigned num) noexcept {
unsigned res = 0;
unsigned bit = 1 << (sizeof(unsigned) * 8 - 2); // The second-to-top bit is set: 1 << 30 for 32 bits
// "bit" starts at the highest power of four <= the argument.
while (bit > num) { bit >>= 2; }
while (bit != 0) {
if (num >= res + bit) {
num -= res + bit;
res = (res >> 1) + bit;
}
else {
res >>= 1;
}
bit >>= 2;
}
return res;
}
각 항의 다음 값을 계산하는 함수는 다음과 같이 간략하게 쓸 수 있다.
void GetSquareRootVals(const unsigned N, const unsigned isqrtN, const unsigned denom, const unsigned minuend, unsigned& aNew, unsigned& denomNew, unsigned& minuendNew)
{
if (isqrtN * isqrtN == N) {
denomNew = 1;
aNew = minuendNew = 0;
}
else {
denomNew = (N - minuend * minuend) / denom;
aNew = (isqrtN + minuend) / denomNew;
minuendNew = aNew * denomNew - minuend;
}
}
모든 내용을 다 갈아넣은(?) 결과는 아래와 같다.
#include <stdio.h>
#include <vector>
using namespace std;
unsigned isqrt(unsigned num) noexcept {
unsigned res = 0;
unsigned bit = 1 << (sizeof(unsigned) * 8 - 2); // The second-to-top bit is set: 1 << 30 for 32 bits
// "bit" starts at the highest power of four <= the argument.
while (bit > num) { bit >>= 2; }
while (bit != 0) {
if (num >= res + bit) {
num -= res + bit;
res = (res >> 1) + bit;
}
else {
res >>= 1;
}
bit >>= 2;
}
return res;
}
void GetSquareRootVals(const unsigned N, const unsigned isqrtN, const unsigned denom, const unsigned minuend, unsigned& aNew, unsigned& denomNew, unsigned& minuendNew)
{
if (isqrtN * isqrtN == N) {
denomNew = 1;
aNew = minuendNew = 0;
}
else {
denomNew = (N - minuend * minuend) / denom;
aNew = (isqrtN + minuend) / denomNew;
minuendNew = aNew * denomNew - minuend;
}
}
struct result {
unsigned a, d, m;
};
bool IsPeriodOdd(const unsigned N, const bool printOddOnly) noexcept {
unsigned sqrN = isqrt(N);
if (sqrN * sqrN == N) {
if (!printOddOnly) {
printf("sqrt(%u) = [%u], period = 0\n", N, sqrN);
}
return false;
}
unsigned a, d, m;
vector<struct result> vResult;
struct result resultN = { sqrN, 1, sqrN };
vResult.push_back(resultN);
for (;;) {
GetSquareRootVals(N, sqrN, resultN.d, resultN.m, a, d, m);
struct result resultT = { a, d, m };
if (vResult.size() > 1 && !memcmp(&vResult[1], &resultT, sizeof(resultT))) {
break;
}
vResult.push_back(resultT);
resultN = resultT;
}
int sz = (int)vResult.size();
if (!printOddOnly || ((sz - 1) & 1)) {
printf("sqrt(%u) = [%u;(", N, vResult[0].a);
for (int i = 1; i < sz; ++i) {
printf("%u", vResult[i].a);
printf((i == (sz - 1)) ? ")" : ",");
}
printf("], period = %d\n", sz - 1);
}
return ((sz - 1) & 1);
}
int main()
{
int count = 0;
for (unsigned i = 1; i <= 10000; ++i) {
if (IsPeriodOdd(i, true)) {
++count;
}
}
printf("odd count: %d\n", count);
}
결과의 일부는 아래와 같다.
sqrt(2) = [1;(2)], period = 1
sqrt(5) = [2;(4)], period = 1
sqrt(10) = [3;(6)], period = 1
sqrt(13) = [3;(1,1,1,1,6)], period = 5
sqrt(17) = [4;(8)], period = 1
sqrt(26) = [5;(10)], period = 1
sqrt(29) = [5;(2,1,1,2,10)], period = 5
sqrt(37) = [6;(12)], period = 1
sqrt(41) = [6;(2,2,12)], period = 3
sqrt(50) = [7;(14)], period = 1
sqrt(53) = [7;(3,1,1,3,14)], period = 5
sqrt(58) = [7;(1,1,1,1,1,1,14)], period = 7
sqrt(61) = [7;(1,4,3,1,2,2,1,3,4,1,14)], period = 11
sqrt(65) = [8;(16)], period = 1
sqrt(73) = [8;(1,1,5,5,1,1,16)], period = 7
sqrt(74) = [8;(1,1,1,1,16)], period = 5
sqrt(82) = [9;(18)], period = 1
sqrt(85) = [9;(4,1,1,4,18)], period = 5
sqrt(89) = [9;(2,3,3,2,18)], period = 5
sqrt(97) = [9;(1,5,1,1,1,1,1,1,5,1,18)], period = 11
...하략...
코드 자체는 단순하기 때문에 굳이 더 최적화할 필요는 없어보인다.
내 PC에서 화면 출력 없이 10,000까지 계산하는데 15ms 정도 소요됐음....
| 음수의 나누기/나머지는 대체 어떻게 해야 되는 걸까? (0) | 2020.11.15 |
|---|---|
| e의 연분수 전개시 특정 수렴값의 분자의 합 (0) | 2020.11.08 |
| endianness 삽질기 (3) | 2020.10.17 |
| n 제곱의 자릿수가 n인 경우의 개수는? (0) | 2020.03.28 |
| 정공법으로 소수 판별 시 더 빠른 방법은? (0) | 2020.03.28 |
